The greatest mathematics has the simplicity and inevitableness of supreme poetry and music, standing on the borderland of all that is wonderful in Science, and all that is beautiful in Art.
H. W. Turnbull
“What is art?” — At many points in the life of an artist, this question will sprout and resonate, possibly inviting to create.
But let’s pose this question to the mathematician, and something even more magical might take life. Or so it is for the case of Christopher Hanusa, professor of mathematics at Queens College of the City University of New York, mathematical artist and wearable art designer.

Christopher creates mathematical art using computational software and brings it to life through 2D and 3D printing methods. The works are often generative, infused with randomness, which is one of the key aspects of Christopher’s work.
When asked the infamous question, Christopher does not limit his answer to the realm of philosophy. But armed with a computer and his mathematical knowledge, and in collaboration with other science artists, he embarked on a quest for an experimental answer, focusing part of his own research on understanding the aesthetics of art. For one of his research projects (with students Christopher Soto and Peter Antonaros), he set up a website where visitors can rate 4000 images of generative art. As the data are coming in, he is working on analyzing the properties that make one piece of generative art better than another.
Christopher commented that his two identities, the professor and the artist, have more in common than one might think: “My life as an artist is intimately connected with my profession as a professor. My role as a professor is to search out the new, explore connections between fields, and share my knowledge and experience with students and the public. I have always used visualizations to understand mathematical concepts in my studies and my research. And I have always found the visual component of explanation important for conveying mathematical truth.”
The art and the research blend at different levels, and they often draw from each other in creative ways. “I take advantage of the precision and beauty of mathematics to present striking geometric forms using bold color schemes. My artwork draws on my varied mathematical interests, including discrete structures and the theory of reflection groups.” — the artist says.
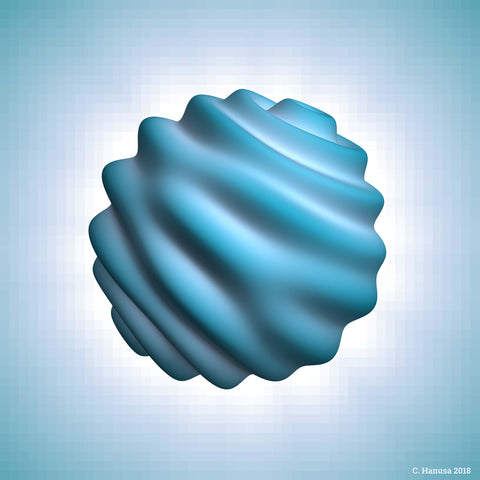
The exploration of the set of all possibilities of a discrete structure is very similar to the exploration of the parameter space of a collection of generative art.
In addition to studying the aesthetics of art, Christopher also researches combinatorics, the study of methods to count discrete objects more effectively, through an experimental approach. For example, Prof. Hanusa computationally generates all possibilities pertinent to a specific combinatory problem and extracts information from them. He then uses this information to conjecture rules that can be proved through mathematical reasoning.
This experimental approach informs his techniques to generate art too: starting from a problem and finding a way to describe it with code, then iterating this experiment until all information is there to create something beautiful.
“When I am creating art, I start with a nugget of inspiration from theoretical mathematics or the physical world and I try to recreate the idea using computer code.” — Says Christopher, indeed — “If it is a mathematical idea, I start with its definition or formula; if I am inspired by something from the physical world, I create an algorithm that manifests the apparent structure. Once I have a working code that faithfully recreates the desired structure, I try to push the idea further.”
It is this “pushing further,” that makes Christopher’s art so unique. The artist breaks barriers, both in math and in art. He would ask himself: “Are there any self-imposed limitations that can be transgressed? Can I modify a particular artistic or aesthetic property yet keep the original ethos? What parameters can I introduce into the code to explore a wide spectrum of possibilities?”
While the art is informed by Prof. Hanusa’s knowledge of multivariable calculus, linear algebra, trigonometry, and geometry, there is also a deeply human aspect that is poured into the work and reflects the artist’s own sense of aesthetics: “Because I integrate randomness into the artwork, not every result is going to be pleasant. Once the code is generating the desired effect, the beauty of a generative framework means that I can rerun the code with new random numbers to generate an alternate version of the piece. I might iterate this multiple times until I see something that catches my eye or I might generate dozens of different variations so that I can find the one that most encapsulates the essence of what I’m going for.”
I take advantage of the precision and beauty of mathematics to present striking geometric forms using bold color schemes, with a key emphasis on the tension between randomness and deliberation.
It is his artistic sensitivity and intuition to determine which direction to take an art piece, in an iterative process, in constant tension between randomness and deliberation. “At each step of this process, there is randomness that helps to inform what the final piece will become, but once the randomness yields something that fits my definition of good, I fix that aspect and develop other aspects of the piece.”
Christopher’s own sense of beauty brought us three series of original sculptures that are part of his solo show “Existence and Uniqueness”, featuring the artist’s iconic bold colors and a variety of inspiration from different realms of reality. The name of the show is inspired by the two properties, existence and uniqueness, that mathematicians have to prove when demonstrating a theorem. These two properties are also embodied in the art itself: the artworks, in their own existence, represent a proof of concept of the mathematical concept that has inspired them; the randomness embedded in their creation guarantees their uniqueness.
The RiemannScapes sculptures, “Misty Steppe,” “Sunset Valley” and “Granite Peak”, are based on Riemann sum, one of calculus techniques to determine the volume of a body, especially useful for volumes of irregular form. “Each RiemannScape sculpture involves breaking up the base into many smaller pieces using a Voronoi diagram and raising each piece to the height of a defining function. Then a generative coloring scheme is added to give the feeling of a landscape. Each piece in the series is entirely unique because the points used to partition the base are randomly generated, the functions determining the heights are unique, and the colors are placed algorithmically based on the points and the function.” says Christopher when explaining the generation of the series.
The Window Evolution sculptures, “Waving Window”, “Pentagonal Window” and “Merging Window”, explore the evolution of an abstract scene in snapshots over time, putting into question our own perception of reality and the flow of time itself. Within a single sculpture, the artist was able to freeze snapshots of shapes that were evolving and changing. “The abstract forms change shape and size as you pass from window to window. You can see each individual frame by looking in from the sides; by looking from one side, you can recreate the entire form in your mind.”
Finally, the Streamlines sculptures, “Divergent Stream” and “Shallot Stream”, describe the streamlines of vector fields. They represent a different take on time and immanence. Think of the direction of wind blowing: that is a vector field. Something light enough to be carried over by the airflow would follow a streamline. The color choice by the artist in this series is very intentional. Not only do the colors complement each to create a beautiful work of art, but they also highlight a physical property of the specific vector fields that are represented: “the dichotomous colors show that equally spaced streamlines at one end arrive in bunches near the other end.”
The solo show also features some of his previous work, in the form of limited edition art prints.

The artist also translates his beautiful art into mathematical jewelry under the brand Hanusa Design. This wearable art is designed using Mathematica and then 3D printed in different materials, including silver and brass through a lost-wax casting method and nylon through a selective laser sintering method and subsequently hand-dyed in bright colors. You can find Hanusa Design Jewelry at hanusadesign.com, and at a variety of museums, galleries, and stores, including the National Museum of Mathematics in New York, NY, and the Exploratorium in San Francisco, CA. Gallery 1064 visitors have a special discount of 10% off all jewelry through March 2022 by entering promo code 1064.
While you enjoy “Existence and Uniqueness” exhibiting at Gallery 1064 starting March 4th, 2022, check more of Christopher Hanusa’s work at the artist’s website, and on his socials (@mathzorro both on Twitter and Instagram). If you want to know more about Christopher, you can also read the full interview here.
Be ready to be launched on a quest of math, art, and beauty.
0 comments


